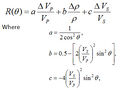Amplitude variations with offset (AVO)
Amplitude Variations with Offset (AVO)
Yuri Nadia Candikia, Ayu Apdila Yuarthi, and Almer Krisnanda Dewantara
AVO has been a prominent technique in detecting hydrocarbons and reducing drilling risk. AVO can detect hydrocarbons because AVO shows the variation of the amplitude of the offset, which represents the amplitude of the wave energy as it passes through the layer which is influenced by the parameters of the speed and density of the coating, so that the density of the layer can be analyzed by analyzing the reflection coefficient. AVO means that amplitude change with offset caused by lithology of fluid. AVO is also known as AVA (amplitude variation with angle) because this phenomenon is based on the relationship between the reflection coefficient and the angle of incidence. But since the angle of incidence affecting the offset and the offset itself can be varied in order to change the angle of incidence (fig.1), so it is commonly known as AVO.
Fig.1. The incident angle is directly proportional to the offset (www.wikiwand.com)
Variation of reflection coefficient is the primary thing in analyzing AVO. It starts when the P-Waves hit the interface, if it is normal incidence (fig.2), the coefficient reflections will be the difference of acoustic impedance (product of density and P velocity) over the sum.
Where RNI is the reflection coefficient.
Fig.2. Normal Incidence (www.ukm.my)
While in the non-normal incidence (fig.3), some of the P-Waves that come will transform into the S waves so that there will be a reflection coefficient variation as a result of different Vp and Vs value. For example, when wave penetrates into a gas layer, the Vp will be decreasing and the Vs will be constant. It means that in certain condition there will be an anomaly in Vp/Vs. This anomaly will cause variation in the reflection coefficient whereas the coefficient itself is the main focus in AVO analysis [1].
Fig.3. Non-normal incidence (Lecture Note Mr. Scott)
The relationship between the reflection coefficient and the angle of incidence in write down the Zoeppritz equations by Karl Zoeppritz since the early 20th century and then this equations was developed again by some figures such as Bortfeld (1961), Aki, Richard and Frasier (1976), Hilterman (1983), and Shuey (1985).
Zoeppritz Equations
Zoeppritz derived the amplitudes of the reflected and transmitted waves using the conservation of stress and displacement across the layer boundary.
Because Zoeppritz equations give four equations with four unknowns, there are some of approximations in order to perform physical interpretation and visualization of the effect of some parameters to reflection coefficient. Some of the approximations are:
a) Bortfeld Approximation (1961)
With assumptions that there is slight change in the layer properties, Bortfeld generates this approximation :
b) Aki, Richard and Frasier Approximation (1976)
Bortfeld approximation then revised by Richard-Frasier (1976) and by Richard-Aki (1980). Approximation of Richard-Frasier is giving a simple equation because it is written in a clear three forms, namely the right-hand side includes the first P wave velocity, the second node density and the last wave velocity.
c) Hilterman (1983)
Hilterman simplified Bortfeld equations by separating the reflection coefficients into the form of acoustic and elastic :
d) Shuey Approximation (1985)
Shuey modified Aki and Richard equation by using variable of poisson ratio as follows:
AVO Classifications
In 1989 Rutherford and Williams introduced a three-fold classification of AVO (amplitude versus offset) characteristics for seismic reflections from the interface between shales and underlying gas sands. The classification scheme they proposed is explicitly defined for gas sands and has become the industry standard; it has proven its validity and usefulness in countless exploration efforts. In 1997 Castagna and Swan proposed AVO crossplotting wherein an estimate of the normal-incidence reflectivity is plotted against a measure of the offset dependent reflectivity. Using this approach Castagna and Swan graphically illustrated the continuum between the classes and defined the characteristics of the classes using what they termed AVO Intercept and AVO Gradient. They also added a class 4 [2].
Fig.4. Classifications of AVO (Lecture Note Mr. Scott)
Class 1 : High impedance Gas-Sandstone
Class 1 sandstone has higher impedance than its cover (shale). Interface between shale and this kind of sandstone will generate a high reflection coefficient and a positive zero offset, but has amplitude magnitude decreasing in order to offset. Class 1 has greater gradient than class 2 and class 3. Sandstone at class 1 is having a change in polarity in certain angle, then the amplitude will be increasing proportionally to the offset.
Class 2 : Near zero impedance contrast gas sandstone
Class 2 sandstone has almost equally acoustic impedance with its cover (seal rock) and the amplitude which is increasing proportionally to the offset. Class 2 sandstone divided into class 2 and class 2p. class 2 sandstone has negative reflection coefficient at zero offset while class 2p has positive at zero offset.
Class 3 : Low impedance gas sandstone
Class 3 has lower acoustic impedance than its cover.
Class 4
Class 4 has negative reflection coefficient at zero offset and amplitude that is decreasing against the offset. There is a change in polarity at a certain angle and then amplitude will increasing proportionally to the offset.
Table 1. Behavior of the various gas sand classes (Lecture Note Mr. Scott)
References :
[1] Choliq, M. T. (2007). Pemodelan parameter elastic batuan degan inversi AVO untuk karakterisasi reservoir hidrokarbon karbonat formasi baturaja, Jawa Barat Utara.
[2] Young, Roger A. and LoPiccolo, Robert D. (2004). Conforming and Non-conforming Sands – An Organizing Framework for Seismic Rock Properties.