Economics: time value of money

Development Geology Reference Manual
Series	Methods in Exploration
Part	Economics and risk assessment
Chapter	The time value of money
Author	Robert S. Thompson
Link	Web page
PDF	PDF file (requires access)

When money is borrowed for a period of time, rent (or interest) for the use of the money must be paid in addition to repayment of the amount borrowed. Thus, money has a time value. In oil and gas property evaluation, two equations with respect to time value are useful: the future value of a lump sum investment and the present value of a lump sum of money received in the future. The concept being applied is one of equivalence, in which the period interest rate is used to calculate this equivalency. In the case when interest is compounded annually, interest earned during each year earns interest in future years. For example, $1.00 invested today at 10% interest compounded annually will be worth $1.10 a year from now, $1.21 two years from now, and $1.33 three years from now. The following equation represents the future value of a lump sum investment compounded annually:

$F=P(1+i)^{t}$

where F is a future lump sum of money, P is a present lump sum of money, i is nominal annual interest rate, and t is time in years. In other words, the amount F received t years from now is equivalent to the present day investment P if the interest rate is i per year.

Present value

Figure 1. Comparison of project cash flows and equivalent present value.

This same equation can be rearranged to solve for the equivalence (or present value) of a future sum of money (such as a project net cash flow) received some time in the future. For example, a dollar that we expect to receive one, two, and three years hence is worth today $0.909, $0.826, and $0.751, respectively, if the time value of money is 10% per year compounded annually. This equation expresses this principle of present value:

$P={\frac {F}{(1+i)^{t}}}$

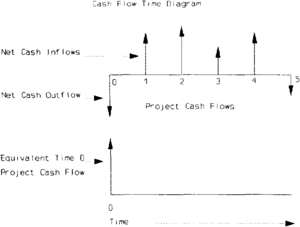
The present value concept is important in petroleum economics because we need to know how to place a value on cash flows to be received from production in future years. This concept is demonstrated in Figure 1. In oil and gas property evaluation, profit is measured in terms of net cash inflows and net cash outflows. In the figure, the horizontal line represents time, the vertical arrows above the horizontal line represent net cash inflows, and the vertical arrows below the horizontal line represent net cash outflows. The profit is usually measured for increments of one year. One exception is the time 0 profit period. Time 0 is the instant in time when the first significant expenditure is made.

When each of the future net cash flows are discounted to time 0 using the present value equation, the resulting net cash flow is called the net present value and is equivalent to the project cash flows at the assumed discount rate. The equivalent time 0 net cash flow is also shown in the figure.

Discount rates

Table 1 shows present value factors at different interest rates (or discount rates) in future years. Some corporations use a discount rate approximately equal to the corporate cost of capital, or the present inflation rate, plus an additional 3-4% (which represents "real" bank interest). At the time this paper was written, this gave an 8-9% corporate discount rate. During this time, however, most U.S. companies were using a discount rate of 12-15%, and international firms were using 15-18%.

Table 1 Present value factors

Years Hence	1%	3%	4%	5%	6%	8%	10%	12%	15%	20%	30%	40%	50%
1	0.990	0.971	0.962	0.952	0.943	0.926	0.909	0.893	0.870	0.833	0.769	0.714	0.667
2	0.980	0.943	0.925	0.907	0.890	0.857	0.826	0.797	0.756	0.694	0.592	0.510	0.444
3	0.971	0.915	0.889	0.864	0.840	0.794	0.751	0.712	0.658	0.579	0.455	0.364	0.296
4	0.961	0.888	0.855	0.823	0.792	0.735	0.683	0.636	0.572	0.482	0.350	0.260	0.198
5	0.951	0.863	0.822	0.784	0.747	0.681	0.621	0.567	0.497	0.402	0.269	0.186	0.132
6	0.942	0.837	0.790	0.746	0.705	0.630	0.564	0.507	0.432	0.335	0.207	0.133	0.088
7	0.933	0.813	0.760	0.711	0.665	0.583	0.513	0.452	0.376	0.279	0.159	0.095	0.059
8	0.923	0.789	0.731	0.677	0.627	0.540	0.467	0.404	0.327	0.233	0.123	0.068	0.039
9	0.914	0.766	0.703	0.645	0.592	0.500	0.424	0.361	0.284	0.194	0.094	0.048	0.026
10	0.905	0.744	0.676	0.614	0.558	0.463	0.386	0.322	0.247	0.162	0.073	0.035	0.017
11	0.896	0.722	0.650	0.585	0.527	0.429	0.350	0.287	0.215	0.135	0.056	0.025	0.012
12	0.887	0.701	0.625	0.557	0.497	0.397	0.319	0.257	0.187	0.112	0.043	0.018	0.008
13	0.879	0.681	0.601	0.530	0.469	0.368	0.290	0.229	0.163	0.093	0.033	0.013	0.005
14	0.870	0.661	0.577	0.505	0.442	0.340	0.263	0.205	0.141	0.078	0.025	0.009	0.003
15	0.861	0.642	0.555	0.481	0.417	0.315	0.239	0.183	0.123	0.065	0.020	0.006	0.002
16	0.853	0.623	0.534	0.458	0.394	0.292	0.218	0.163	0.107	0.054	0.015	0.005	0.002
17	0.844	0.605	0.513	0.436	0.371	0.270	0.198	0.146	0.093	0.045	0.012	0.003	0.001
18	0.836	0.587	0.494	0.416	0.350	0.250	0.180	0.130	0.081	0.038	0.009	0.002	0.001
19	0.828	0.570	0.475	0.396	0.331	0.232	0.164	0.116	0.070	0.031	0.007	0.002	0.000
20	0.820	0.554	0.456	0.377	0.312	0.215	0.149	0.104	0.061	0.026	0.005	0.001
21	0.811	0.538	0.439	0.359	0.294	0.199	0.135	0.093	0.053	0.022	0.004	0.001
22	0.803	0.522	0.422	0.342	0.278	0.184	0.123	0.083	0.046	0.018	0.003	0.001
23	0.795	0.507	0.406	0.326	0.262	0.170	0.112	0.074	0.040	0.015	0.002
24	0.788	0.492	0.390	0.310	0.247	0.158	0.102	0.066	0.035	0.013	0.002
25	0.780	0.478	0.375	0.295	0.233	0.146	0.092	0.059	0.030	0.010	0.001
26	0.772	0.464	0.361	0.281	0.220	0.135	0.084	0.053	0.026	0.009	0.001
27	0.764	0.450	0.347	0.268	0.207	0.125	0.076	0.047	0.023	0.007	0.001
28	0.757	0.437	0.333	0.255	0.196	0.116	0.069	0.042	0.020	0.006	0.001
29	0.749	0.424	0.321	0.243	0.185	0.107	0.063	0.037	0.017	0.005
30	0.742	0.412	0.308	0.231	0.174	0.099	0.057	0.033	0.015	0.004
31	0.735	0.400	0.296	0.220	0.164	0.092	0.052	0.030	0.013	0.004
32	0.727	0.388	0.285	0.210	0.155	0.085	0.047	0.027	0.011	0.003
33	0.720	0.377	0.274	0.200	0.146	0.079	0.043	0.024	0.010	0.002
34	0.713	0.366	0.264	0.190	0.138	0.073	0.039	0.021	0.009	0.002
35	0.706	0.355	0.253	0.181	0.130	0.068	0.036	0.019	0.008	0.002
36	0.699	0.345	0.244	0.173	0.123	0.063	0.032	0.017	0.007	0.001
37	0.692	0.335	0.234	0.164	0.116	0.058	0.029	0.015	0.006	0.001
38	0.685	0.325	0.225	0.157	0.109	0.054	0.027	0.013	0.005	0.001
39	0.678	0.316	0.217	0.149	0.103	0.050	0.024	0.012	0.004	0.001
40	0.672	0.307	0.208	0.142	0.097	0.046	0.022	0.011	0.004	0.001
41	0.665	0.298	0.200	0.135	0.092	0.043	0.020	0.010	0.003	0.001
42	0.658	0.289	0.193	0.129	0.087	0.039	0.018	0.009	0.003
43	0.652	0.281	0.185	0.123	0.082	0.037	0.017	0.008	0.002
44	0.645	0.272	0.178	0.117	0.077	0.034	0.015	0.007	0.002
45	0.639	0.264	0.171	0.111	0.073	0.031	0.014	0.006	0.002
46	0.633	0.257	0.165	0.106	0.069	0.029	0.012	0.005	0.002
47	0.626	0.249	0.158	0.101	0.065	0.027	0.011	0.005	0.001
48	0.620	0.242	0.152	0.096	0.061	0.025	0.010	0.004	0.001
49	0.614	0.235	0.146	0.092	0.058	0.023	0.009	0.004	0.001
50	0.608	0.228	0.141	0.087	0.054	0.021	0.009	0.003	0.001

Some corporations advocate calculating an average reinvestment opportunity rate based on past performance and using this discount rate. When the historical record is analyzed (sometimes called a post audit), the analysis can be done on both a dollars-of-the-day basis and in terms of constant purchasing power dollars. It is not an easy task to put all the project cash flows in terms of constant purchasing power for the "basket of goods" the corporation purchases. The concept of using the future price or cost increases of the capital goods purchased by the corporation as an index for loss of purchasing power is discussed in a paper by Krasts and Henkel, in which they apply the concept to discounted cash flow rate of return (DCFROR) calculations. When the post audit average constant purchasing power rate of return is used as the discount rate in net present value calculations, the effect is that projects are compared assuming treasury growth. The project cash flows must also be in terms of constant purchasing power.

This brief discussion demonstrates the diverse approaches that are used to estimate the proper discount rate. This topic deserves more research. For example, should multiple discount rates be used--one discount rate for high risk exploration projects versus a lower discount rate for lower risk development projects? At issue here is how to best deal with risk. A good case can be made for using expected values (probabilistic approach) to account for risk and using the discount rate to account for the time value of money.) This method is strongly recommended by the author.

The "Rule of 72" is a useful rule of thumb that allows us to estimate the doubling time or rate of any proposed investment. To find the doubling time of a sum invested at any compounded interest rate, divide the interest rate into 72. For example, at 12% compounded annual interest, the investment will approximately double in six years.

More detailed discussions of the time value of money can be found in Thompson and Wright.

See also

• Risk: expected value and chance of success
• Cash flow model
• Taxes
• Economics: key parameters

discounted cash flow rate of return