Economics: key parameters

Development Geology Reference Manual
Series	Methods in Exploration
Part	Economics and risk asseement
Chapter	Key economic parameters
Author	Peter R. Rose, Robert S. Thompson
Link	Web page
Store	AAPG Store

Each of the many economic parameters used in assessing and comparing oil and gas ventures has utility in measuring some desired aspect of the proposed opportunity. Unfortunately, no single parameter “does it all,” but some are better than others. For a thorough treatment of this subject, see Capen et al., Newendorp, Megill, and Thompson and Wright. The yardsticks discussed below represent the more commonly used economic measures. The key economic parameters for the example development well and the example multiwell extension project, given in the article Building a cash flow model, are summarized here in Tables 1 and 2, respectively.

Table 1 Summary of key economic parameters for example development well

Economic Parameter	Value
NPV at 4%	cost::1,015,600 USD
DCFROR (%)	44.9
Cumulative undiscounted NCF (after-tax)	cost::1,201,893 USD
Undiscounted payout (years)	1.0
Undiscounted profit to investment ratio	cost::1,201,893 USD/1,375,000 = 0.87
Investment efficiency (4% discount rate)	cost::1,015,600 USD/1,375,000=0.74
Discounted profit to investment ratio (4% discount ratio)	cost::1,015,600 USD/1,375,000=0.74
Expected net present value (4% discount rate) Ps = 0.80, Pf = 0.20	0.80 × 1,015,600 – 0.20 × 495,000 = cost::713,480 USD

Table 2 Summary of key economic parameters for example multiwell extension project

Economic Parameter	Value
NPV at 4%	cost::2,729,760 USD
DCFROR (%)	28.0
Cumulative undiscounted NCF (after-tax)	cost::3,634,721 USD
Undiscounted payout (years)	3.4
Undiscounted profit to investment ratio	cost::3,634,721 USD/7,225,000 = 0.50
Investment efficiency (4% discount rate)	cost::2,729,760 USD/2,429,333 = 1.12
Discounted profit to investment ratio (4% discount ratio)	cost::2,729,760 USD/6,859,396 = 0.40
Expected net present value (4% discount rate) Ps = 0.70, Pf = 0.30	0.70 × 2,729,760 – 0.30 × 990,000 = cost::1,613,832 USD

Annual budgeting is carried out once the drilling program is chosen by selecting projects that rank high with regard to whichever economic yardsticks best fit the organization's needs.

Net present value

Net present value (NPV), or net present worth, is based on the concept of equivalence discussed in the article Economics: time value of money. The net present value is equivalent to the future cash flows at the assumed discount rate. It is the fundamental parameter to express value of a project assuming success, and it measures the cumulative cash worth of the venture above the corporate discount rate. Ordinarily, it is based upon the mean reserves case. For the development well in Table 1 of the article Building a cash flow model, the NPV at 4% = cost::1,015,600 USD. For the extension project (see Table 3 of Building a cash flow model), the NPV at 4% = cost::2,729,760 USD.

Table 3 Comparison of discounted profit to investment ratio and investment efficiency ratio

Time	NOI	Invest.	NCF	Neg. NCFs	PV Factor @4%	PV of Neg. NCFs	PV of Invest.	PV of NCFs	Cum. Disc. NCF @4%
0		1000	–1000	–1000	1.0000	–1000.0	1000.0	–1000.0	–1000.0
1	600	1000	–400	–400	0.9615	–384.6	961.5	–384.6	–1384.6
2	800	200	600		0.9246	0.0	184.9	554.8	–829.8
3	1100	0	1100		0.8890	0.0	0.0	977.9	148.1
					NPV	–1384.6	2146.4	148.1

Discounted cash flow rate of return

Figure 1 Present value profile and determination of DCFROR for example development well and example multiwell extension project.

Discounted cash flow rate of return (DCFROR), or internal rate of return, is calculated by a trial-and-error method. In this method, different discount rates—and thus present value (PV) factors—are inserted in the cash flow model to yield a series of NPVs, beginning with a discount rate of zero (equals undiscounted net cash flow stream). For project cash flows, such as our two examples, the cumulative NPV of a project will decline with successively higher discount rates (Figure 1). The point at which the declining curve intersects the zero present value line corresponds to the DCFROR of the project. For complex projects involving subsequent enhanced oil recovery additions, the resulting dual rate problem may generate more than one DCFROR.

If treasury growth is the goal of the firm, selecting projects based on DCFROR will not necessarily result in the best selection of projects. Thompson and Wright discuss the use of DCFROR as a decision criterion and the reinvestment assumption.

It is the authors' opinion that DCFROR should not be used as a “risking measure”—this measure has nothing whatever to do with project risk! Some firms think (incorrectly) that by setting high DCFROR hurdle rates, they are selecting the better projects. Ideally, such hurdle rates should reflect the current real earning performance of the firm. For a discussion of the use of high hurdle rates to account for risk, see Thompson and Wright. Excessively high DCFROR hurdles in fact tend to favor short-term, lower reserve, high profit projects (which, upon project completion, the company has a hard time replacing) at the expense of long-term, larger reserve projects—the kind of projects that build corporations. DCFROR is only recommended as a minimum hurdle that all proposed projects must clear to be considered.

The present value profile for the two example cases (Figure 1) shows graphically that the DCFROR for the development well is approximately 45% and that the DCFROR for the extension project is approximately 28%. These two DCFRORs demonstrate an important point about development well economics and exploration economics. Development wells are evaluated on an incremental basis. If over the long term the firm is to generate sufficient cash flow from production to sustain a continuing exploratory program, the DCFROR for the development well must be greater than the DCFROR for the exploration project. This is true because the development well must (1) earn enough to pay for itself, (2) earn a satisfactory return as an investment, and (3) provide additional earnings proportionally equivalent to at least the true cost of the exploratory effort required to discover it (including exploratory dry holes).

Finally, in capital budgeting, management must remember that exploration and prospect generation are long-term commitments. The capital commitment for exploration should not be turned on and off from year to year. Considerable lead time is required for geological and geophysical studies. One measure of the success of an exploratory program is by a historical analysis of past exploration activities including the actual development expenditures. The result should be a DCFROR greater than the minimum hurdle rate. On the other hand, a historical analysis of the development costs and subsequent production should result in higher historical DCFRORs than the total exploration program since the exploratory costs would be left out in this analysis.

Payout

Figure 2 Undiscounted and discounted cumulative net cash flow streams for example multiwell extension project.

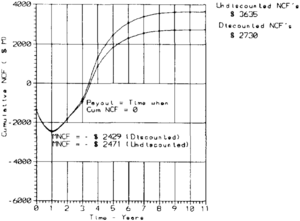
The payout is the length of time required for the venture to generate income sufficient to equal capital investment and expenses (see Figure 2). This measure is of greater importance to small investors, who are concerned about liquidity and risk exposure. It can be calculated using constant purchasing power dollars. In cases where a loan payment is required, dollars of the day should be used. Its major drawbacks are that it doesn't consider cash flows after payout occurs, nor does it address any aspect of investment performance. Although payout implicitly touches on financial risk and exposure (which translates to “when do I get my money back?!”), it does not address chance of success in any way.

Megill points out a useful rule of thumb: a rough, reciprocal relationship exists between payout and DCFROR. A project that pays out in 3 years will have a DCFROR of about 33%; one that pays out in 4 years will have a DCFROR of about 25%.

Individual development wells should pay out in less than 3 years. The development well in Table 1 in Building a cash flow model pays out in approximately 1 year. Most exploration projects should pay out in about 4 to 8 years, except for very large projects in difficult areas. The development well example has a 1.0 year payout, whereas the extension project has a 3.4 year payout.

Discounted payout, in which both the investment stream and the revenue stream are discounted, is probably a slightly more useful measure, but is not widely employed. The extension project has a discounted payout of approximately 3.5 years.

Maximum negative cash flow

Maximum negative cash flow (MNCF) is an important measure because it expresses the greatest cumulative out-of-pocket expense—that is, the greatest cash “exposure” in any project—and thus is useful in budgeting, planning, and project comparison in firms that are cash constrained. It is derived from the cash flow model by expressing the net of investments and costs against early revenues. Thus, it is the turnaround spot on the cumulative net cash flow stream. It is not a discounted number. The undiscounted MNCF for the extension project is cost::2,471,507 USD, as shown in Figure 2 (see Table 3 in Building a cash flow model).

Like payout, MNCF may be relatively more important to risk-averse smaller investors. It does not address chance of project success. For individual development wells, such as our example problem, MNCF is not particularly useful since there is rarely much overlap in time between capital expenses and production revenue.

Undiscounted profit to investment ratio

Undiscounted profit to investment ratio (P/I) measures the magnitude of cash flow with respect to investment, but it does not address the time frame in which the profits are received. Neither does it express the magnitude of the venture or any aspect of risk. Profit can be defined as the net operating income (NOI) or as the net cash flow (NCF). If profit is defined as NCF, the minimum acceptable ratio is 0.0, whereas if it is defined as NOI, the minimum acceptable ratio is 1.0. The difference between the two ratios for a project is always 1.0. If someone uses profit to investment ratios, ask them what their definition of profit is. Also, projects need to be ranked and compared on a consistent basis.

The undiscounted profit to investment ratio (with profit as NCF) for the development well and the extension project in Building a cash flow model are cost::1,201,893 USD/cost::1,375,000 USD = 0.87 and cost::3,634,721 USD/cost::7,225,000 USD = 0.50, respectively.

Investment efficiency

Several economic parameters can be used to measure investment efficiency. When capital is limited, these yardsticks permit projects to be ranked from high to low until the available capital is exhausted. The investment efficiency ratios are indicators of the projects profit per dollar of investment. The two parameters presented here are very similar but do differ slightly. Primarily, they differ in our perception of how the budgeting process actually takes place. Regardless of the economic yardstick used, you must be consistent and compare all projects with the same yardstick. The first ratio is called the investment efficiency and is defined as

${\mbox{Investment efficiency}}\,=\,{\frac {\mbox{Cumulative net present value}}{\mbox{PV of maximum negative cash flow}}}$

The second parameter is called the discounted profit to investment ratio and is defined as follows:

${\mbox{Discounted profit to investment ratio}}\,=\,{\frac {\mbox{Cumulative net present value}}{\mbox{PV of all investments}}}$

Note that the numerator is the same for both yardsticks. However, the investment efficiency parameter uses only the present value of the early negative NCFs in the denominator. The present value of the MNCF is defined in this case as the greatest cumulative discounted out-of-pocket expense. The discounted profit to investment ratio keeps all investments separate and uses the cumulative present value of each investment as the denominator. The significance of this difference has not been rigorously tested to our knowledge. It is believed, however, that the investment efficiency can be best applied when the investor believes that the project itself finances some or all of future project investments, in contrast to the case when it is felt that all future investments must go through the budgeting process and compete with other projects for investment capital. In the case of smaller projects that generate cash rapidly, the investment efficiency parameter may be the best one. In cases where major capital expenditures occur over several years, however, the profit to investment ratio may be more representative of the actual process. Regardless of which parameter you use, be consistent!

Although these are highly recommended yardsticks, they do have some limitations since they do not express the magnitude of cash flows or the chance of success. Table 3 shows a simple example demonstrating the difference in the two economic yardsticks.

The investment efficiency for our example development well is cost::1,015,600 USD/cost::1,375,000 USD = 0.74, whereas the investment efficiency for the extension project is cost::2,729,760 USD/cost::2,429,333 USD = 1.12. Both ratios are based on a 4% discount rate.

Expected net present value and ENPV to expected investment ratio

Figure 3 Expected value profile plot. Expected value is plotted versus probability of success example for development well and multiwell extension project.

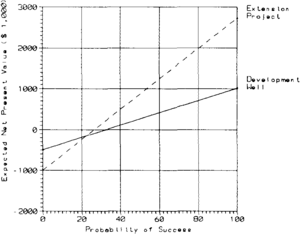
The expected net present value (ENPV) and the expected net present value to expected investment ratio are two highly recommended economic parameters for comparing and ranking projects, primarily because these concepts take into account the uncertainty and help in predicting outcomes of program inventories. They are particularly useful as exploration measures and less so for development projects.

The ENPV considers the net after-tax monetary value of the venture over the full life of the project (gross revenues minus capital investments and costs) discounted at the corporate discount rate. It incorporates current views on future wellhead prices, costs, and inflation rates and also takes into account the probabilities of success and failure as well as the cost of failure. Thus, it is a “risked” value and is the amount the company could expect to make on average if this prospect (or ones like it) could be undertaken many times.

If the development well modeled in Table 1 in Building a cash flow model had been assigned an 80% chance of success by the geologist, the calculated ENPV (aftertax) at 4% would be cost::713,480 USD (0.80 × cost::1,015,600 USD – 0.20 × cost::495,000 USD). Since this is a linear function, a plot of ENPV versus the probability of success results in a straight line. Assume two points (P_s = 1 and 0) to define the line. The intersection where the ENPV is zero will give the breakover point for the probability of success. Figure 3 demonstrates this concept. The breakover probability of success for the development well is approximately 33%.

If the extension project had been assigned a 70% chance of success by the geologist, the calculated ENPV (after-tax) at 4% would be cost::1,613,832 USD (0.70 × cost::2,729,760 USD – 0.30 × cost::990,000 USD). The breakover probability of success for the extension project is approximately 27% (see Figure 3).

The expected net present value is useful in comparing large or complex ventures, as well as projects having different discovery probabilities and reserves potential. The parameter is useful in prospect inventories and for program planning and budgeting if estimates of reserves and discovery probability are reliable. The expected net present value to expected investment ratio is also useful in capital investment decisions when capital is limited. Newendorp) demonstrates the use of this investment efficiency ratio.

Again, a final cautionary note: do not carry out economic analyses—that is, cash flow models—on expected (or “risked”) reserves. The risking is done only on the net present value of the “success case”!

See also

• Risk: expected value and chance of success
• Cash flow model
• Taxes
• Economics: time value of money
• Risk: dealing with risk aversion
• Economics: fundamental equations for oil and gas property evaluation
• Economics of property acquisitions
• Uncertainties impacting reserves, revenue, and costs

External links

find literature about Economics: key parameters

• Original content in Datapages
• Find the book in the AAPG Store

net present value

discounted cash flow rate of return

net cash flow

maximum negative cash flow

expected net present value